You have a horizontal grindstone (a disk) that is 86 kg, has a 0.38 m radius, is turning at 88 rpm (in the positive direction), and you press a steel axe against the edge with a force of 18 N in the radial direction.

Assuming that the kinetic coefficient of friction between steel and stone is 0.20, calculate the angular acceleration of the grindstone in rad/s².

What is the number of turns, N, that the stone will make before coming to rest?

Answer:

Given the mass of the grind stone,m = 86 Kg

The radius of the stone r =0.38 m

The moment of inertia of the stone disk,I =1/2 mr²

I = 1/2 . 86*0.38² = 6.21 Kgm²

A.

The initial rotation per min = 88 rpm

So the initial angular velocity, w = 9.21 rad/s

The force by friction,F = 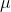 *N

where 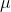 = 0.2 and N = normal force = 18 N

So F = 0.2*18 = - 3.6 N ( negative because it is in opposite direction)

The torque given by friction T = F×r = -3.6*0.38

Torque T = - 1.37 Nm

Also we have the equation of torque T = I a

Where a is angular acceleration.

Or a = T/I = -1.37/6.21 = - 0.22 rad/s²

So the angular acceleration of the stone = -0.22 rad/s²

B.

According to law of conservation of energy, the change in rotational Kinetic energy = work done by friction

0- 1/2 I w² = T* d

Where d is the angular displacement

d = Iw²/2T = 6.21*9.21²/2*1.37 = 192.24 rad.

Or 192.24/2π = 30.6 Turns.